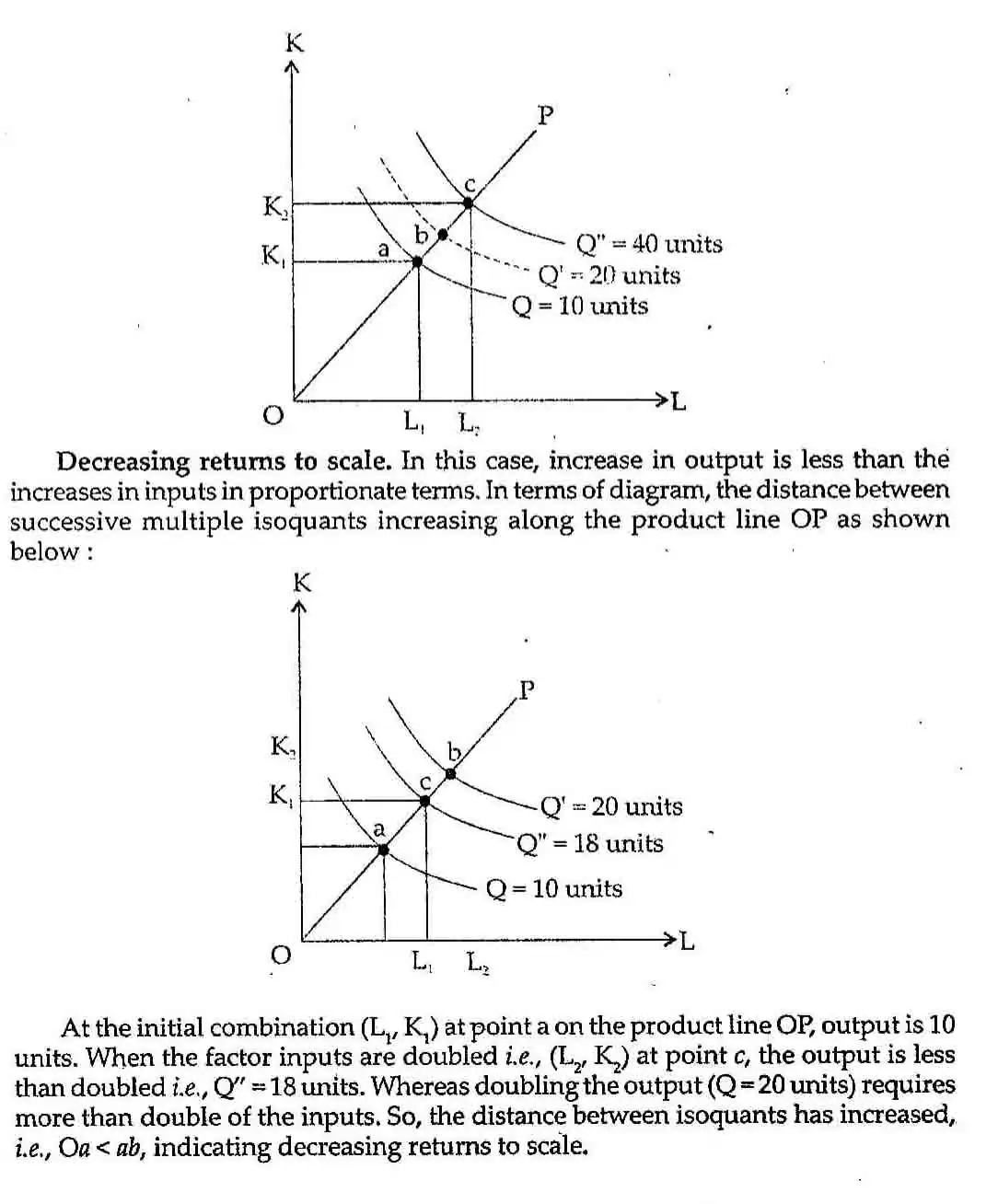
Explain the concept of Returns to Scale. Use diagrams.
Returns to a Scale:
When all factors are increased simultaneously and ultimately the production of output also changes. Concept of returns of returns to scale is associated with the tendency of production that is observed when the ratio between the factors is kept constant but the scale is expanded.
When all the factors are increased in the conditions of constant techniques, three production possibilities arise:
- Output increases in the same proportion as the increase in the amounts of the factors of production —Constant Returns to Scale.
- Output increases in a greater proportion as compared to the increase in the amounts of factors of production — Increasing Returns to Scale.
- Output increase in a smaller proportion as compared to the increase in the amounts of the factors of production — Decreasing Returns to Scale.
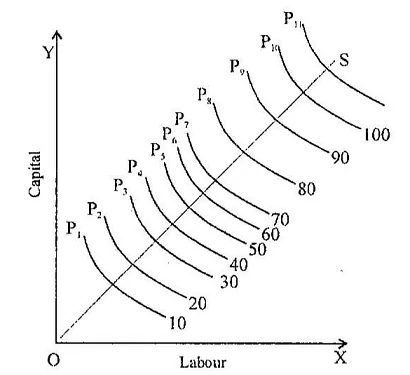
In the figure P1, P2, P3….P10 are isoquants. They show 10, 20, 30…, 100 units of output respectively. OS is the scale line which is cut by the isoquants
at unequal distances.
Initially, the distance between two consecutive isoquants is small, it then becomes constant, and ultimately increases. It shows that in the first stage when the scale is expanded returns increase, in the second stage they become constant & in the third and last stage, they decrease. It can be seen that Oa > ab > bc > cd.
It means that to enable the firm to rise from isoquants P1 to P2 (so that production increases from 10 to 20 units), the amount of factors required is less than the amount required to produce the initial 10 units of output. This position seems to hold true till P4 representing increasing returns to scale.
When the firm goes from curve P4 to P5 to P6, constant returns to scale are obtained as cd = de = ef on the scale line indicates this. After this, the increasing distance between two consecutive isoquant is an indication that to obtain the same increase in output, factors will have to be increased at a higher and higher rate. On the scale line OS, fg < gh < hi < ij indicate this concept of decreasing returns to scale.
The laws of returns to scale refer to the long run analysis of production. In the long run all the factors become variable. So output can be expanded by changing all the factors simultaneously, so that the scale of production is changed. The term returns to scale refers to the situation of increase in output by increasing all the factors by the same proportion. Firms can choose any scale of production. They can double or triple output or go out of business completely.
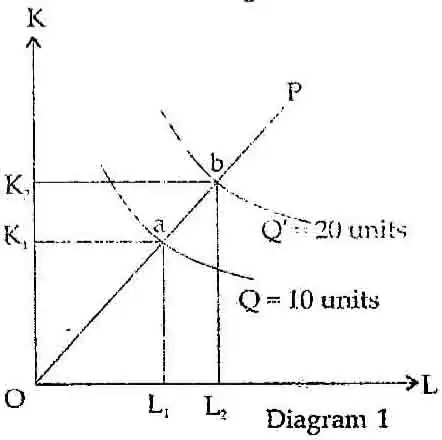
Return to scale can be constant, increasing or decreasing in the long run. Constant returns to scale. Technically, the term constant returns, means that the quantitative relationship between input and output stays constant or the same, when output is increased. If a firm doubles its inputs, it doubles output; if it triples inputs, it triples output; and so forth. Furthermore, if input prices are fixed, constant returns implies that the average cost of production does not change with scale.
In terms of following diagram 1, in constant returns to scale, the isoquants are equidistant along the product line OP, where product line is defined as a line showing movement from one isoquant to another resulting from the change in factor input combinations. In this case, by doubling the capital and labour inputs, the firm is able to double the output at level of 20 units. The distance between consecutive multiple isoquants remains the same under constant returns to scale i.e., Oa = ab.
Increasing returns to scale. Technically, the phrase increasing returns to scale refers to the relationship between inputs and outputs. When we say that a production function exhibits increasing returns, we mean that given percentage increase in inputs leads to a larger percentage increase in the production of output.
For example, if a firm doubled or tripled inputs, it would more than double or triple output. When the prices of inputs do not change with output levels, then the increasing returns to scale also means that as output rises, average cost of production falls. In terms of the following diagram 2, increases returns to scale implies that the distance between consecutive multiple decreases along the product line i.e., Oa < ab.